The basic algebraic operations can be performed on polynomials of different types. These four basic operations on polynomials can be given as,

• Addition of polynomials
• Subtraction of polynomials
• Multiplication of polynomials
• Division of polynomials

Addition of Polynomials

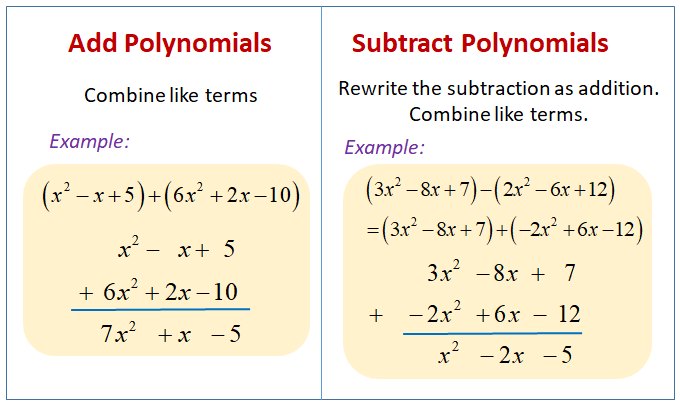
Addition of polynomials is one of the basic operations that we use to increase or decrease the value of polynomials. Whether you wish to add numbers together or you wish to add polynomials, the basic rules remain the same. The only difference is that when you are adding, you align the appropriate place values and carry the operation out. However, when dealing with the addition of polynomials, one needs to pair up like terms and then add them up. Otherwise, all the rules of addition from numbers translate over to polynomials. Have a look at the image given here in order to understand how to add any two polynomials.

Example: Find the sum of two polynomials: 5x³+3x²y+4xy−6y², 3x²+7x²y−2xy+4xy²−5

Solution:

First, combine the like terms while leaving the unlike terms as they are. Hence,

(5x³+3x²y+4xy−6y²)+(3x²+7x²y−2xy+4xy²−5)

= 5x³+3x²+(3+7)x²y+(4−2)xy+4xy²−6y²−5

= 5x³+3x²+10x²y+2xy+4xy²−6y²−5

Subtraction of Polynomials

As discussed above, the rules for the subtraction of polynomials are very similar to subtracting two numbers. To subtract a polynomial from another, we just add the additive inverse of the polynomial that is being subtracted to the other polynomial. Another easy way to subtract polynomials is, just change the signs of all the terms of the polynomial to be subtracted and then add the resultant terms to the other polynomial as shown below. We just have to align the given polynomials based on the like terms.

Example: Find the difference of two polynomials: 5x³+3x²y+4xy−6y², 3x²+7x²y−2xy+4xy²−5

Solution:

First, combine the like terms while leaving the unlike terms as they are. Hence,

(5x³+3x²y+4xy−6y²)-(3x²+7x²y−2xy+4xy²−5)

= 5x³-3x²+(3-7)x²y+(4+2)xy-4xy²−6y²+5

= 5x³-3x²-4x²y+6xy-4xy²−6y²+5